The Milkovic pendulum device incorporates mechanical "earthing" which prevents the hammer side falling for most of the pendulum swing. By so doing it introduces the same kind of asymmetry to the action of the Newtonian Gravitational wind as the Keenie.
I have spent a considerable time over the last few weeks wondering how I could arrange for feedback of energy from the hammer side to the pendulum side so as to obtain continuous running - or at least a Proof of Principle.
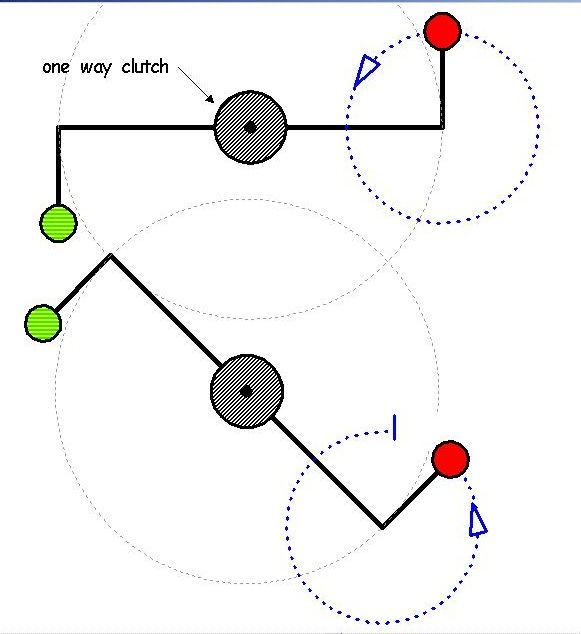
In the first place it is clear that a 360° pendulum is superior than a Milkovic's smaller arc pendulum since it is clear when reset has been achieved, i.e. when the pendulum returns to its 0° position.
Also the amount of feedback needed to obtain reset can be reduced to a very small value by reducing the friction at the bearing and the air resistance of the pendulum bob and shaft. I suppose the ultimate limit would be a magnetic bearing at the pivot and a hard vacuum enclosure.
By contrast the reset of the Keenie falling weight is limited by the coefficient of elastic impact between the single falling weight and the compound pendulum made up of the remaining weights.
------------------------------
Eventually I realised that neither feedback nor extraordinary improvements of the pendulum are required since the action of the 360° pendulum in rotating the system as a whole leads to divergence between the relative and absolute reset angle. This is best understood from studying the two diagrams above.

