Hypothesis .. Raising GPE without using Law of Levers ? ..
Moderator: scott
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
I approach energy differently than the conventional view. I don’t subscribe to the idea of conservation of energy in a closed system. One of my ideas is that Time is the ultimate form of energy, underpinning all transformations and interactions.
Perhaps the universe itself acts as an energy well—a reservoir where all energy is ultimately conserved. From this perspective, even the energy we observe as lost or transformed might be 'stored' in the fabric of time or space, aligning with principles like E = mc^2 where energy and matter are deeply interconnected.
This idea is still a work in progress—I’m struggling to conceptualize or express it fully. Could time be the fundamental mechanism through which this universal energy balance is maintained? How might time's flow connect to the energy transformations we observe, and what does that mean for closed systems or conservation laws?
That doesn’t mean I know how to pull energy out of space-time to add energy to a closed system; far from it. But the idea intrigues me: if time is a form of energy, could there be mechanisms we haven’t yet discovered to tap into it?
For instance, we already know energy and mass are deeply connected through E = mc^2, and that space-time itself is dynamic, capable of bending, stretching, and interacting with matter and energy. Could there be ways in which the fabric of space-time plays a role in sustaining or even supplementing energy within a system?
I don’t have answers, just dumb questions.
Perhaps the universe itself acts as an energy well—a reservoir where all energy is ultimately conserved. From this perspective, even the energy we observe as lost or transformed might be 'stored' in the fabric of time or space, aligning with principles like E = mc^2 where energy and matter are deeply interconnected.
This idea is still a work in progress—I’m struggling to conceptualize or express it fully. Could time be the fundamental mechanism through which this universal energy balance is maintained? How might time's flow connect to the energy transformations we observe, and what does that mean for closed systems or conservation laws?
That doesn’t mean I know how to pull energy out of space-time to add energy to a closed system; far from it. But the idea intrigues me: if time is a form of energy, could there be mechanisms we haven’t yet discovered to tap into it?
For instance, we already know energy and mass are deeply connected through E = mc^2, and that space-time itself is dynamic, capable of bending, stretching, and interacting with matter and energy. Could there be ways in which the fabric of space-time plays a role in sustaining or even supplementing energy within a system?
I don’t have answers, just dumb questions.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Not sure I understand your view on this. You have translational energy, and rotational energy. The hoop has more inertia, because its mass is distributed further from its axis, so it is harder to spin up: therefor harder to slow down as well. Because of this, it is moving slower at the bottom of the ramp.My biggest point is missing though. That is if I stopped the hoop just before impact at the flags at the bottom where is the missing energy? Where is it physically? Energy in a closed system is conserved. There is no "energy well" to put it in under todays physics. It is not all spinning. It is just missing due to V^2 term.
If you rolled all the rollers down a half pipe, in an ideal world, they are all going to roll back up the other side to (nearly) the same height, even though the hoop attains a slower maximum velocity at the bottom compared to the sphere. I don't see any energy lost here.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
I agree .. here I made a sim of a disk verses a ring ( attached below ) - both have mass of 10 kgs and roll down a slope of 20 degrees losing 6 meters of vertical height .. the sim pauses when each gets to the -6 meters mark .. look at the Outputs for velocity and KE ( RKE and TKE ) for each when paused ..Tarsier79 wrote:Not sure I understand your view on this. You have translational energy, and rotational energy. The hoop has more inertia, because its mass is distributed further from its axis, so it is harder to spin up: therefor harder to slow down as well. Because of this, it is moving slower at the bottom of the ramp.My biggest point is missing though. That is if I stopped the hoop just before impact at the flags at the bottom where is the missing energy? Where is it physically? Energy in a closed system is conserved. There is no "energy well" to put it in under todays physics. It is not all spinning. It is just missing due to V^2 term.
If you rolled all the rollers down a half pipe, in an ideal world, they are all going to roll back up the other side to (nearly) the same height, even though the hoop attains a slower maximum velocity at the bottom compared to the sphere. I don't see any energy lost here.
Total KE is slightly different because of dissipative losses to frictions etc, and skipping and slippage etc - T79's scenario of running them back up the half pipe would see both nearly gain their original heights and GPE ( but not both exactly the same but near enough imo, small percentage difference ) ..
..................
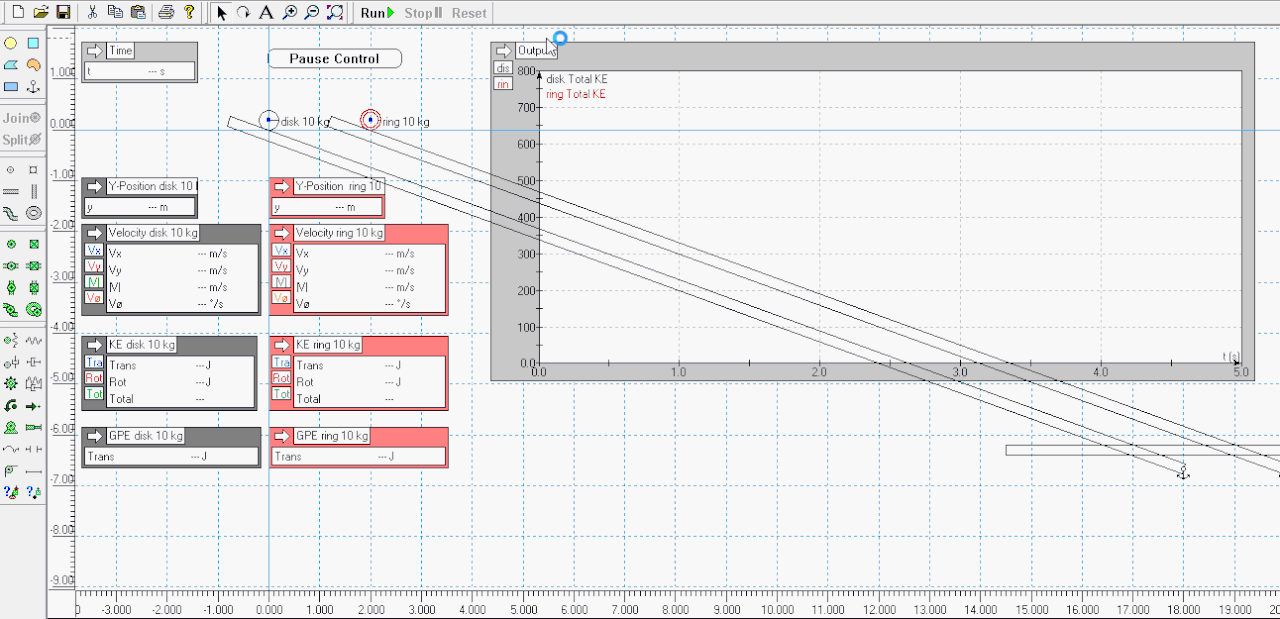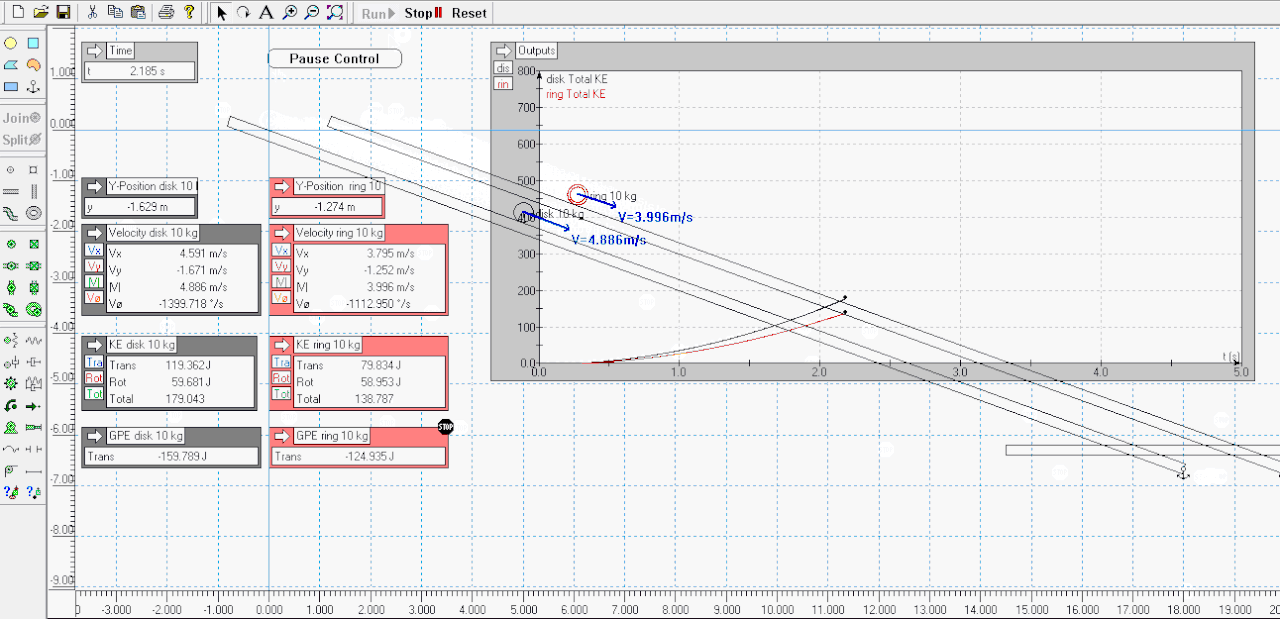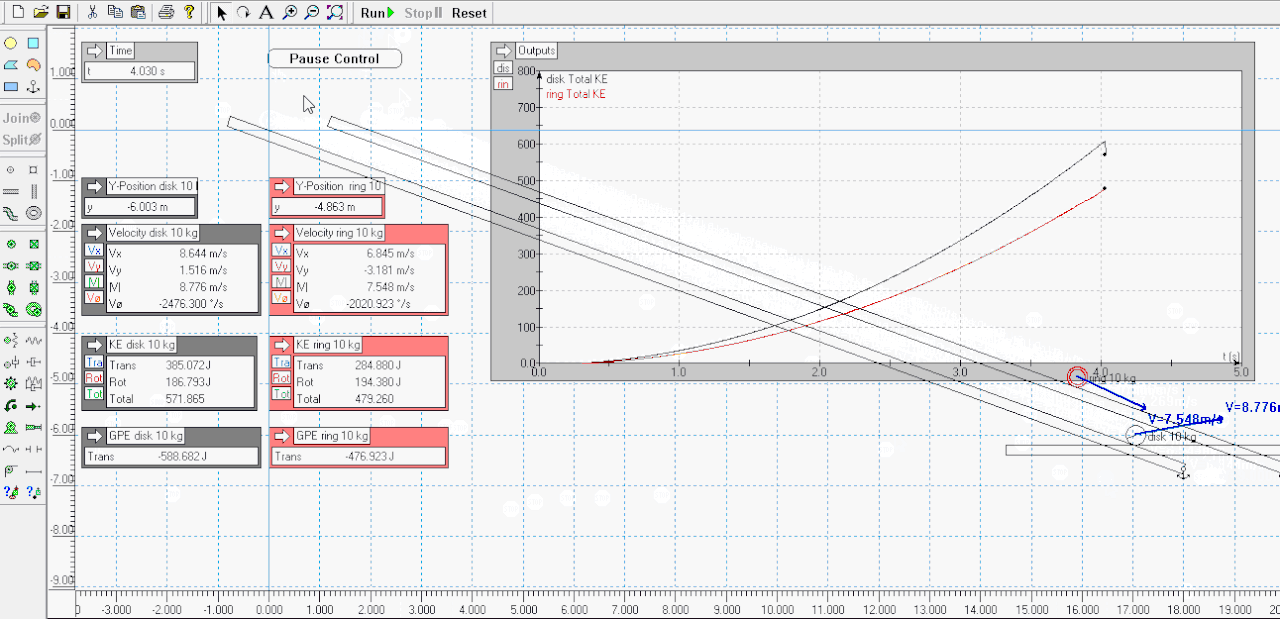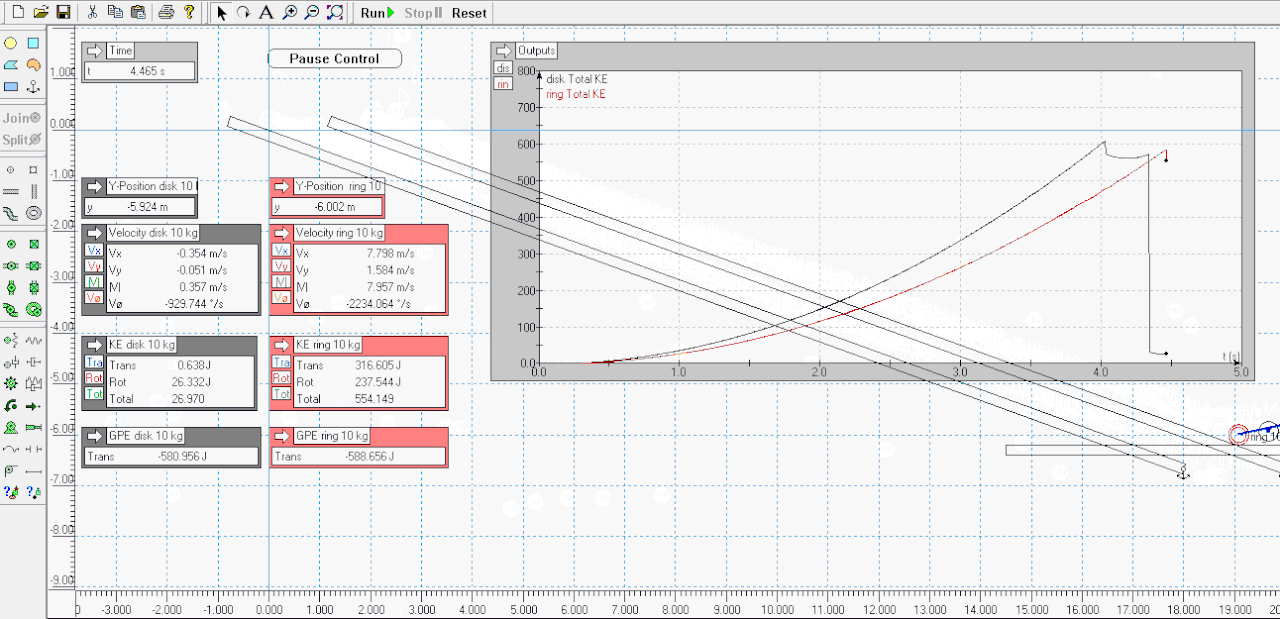
..................
- Attachments
-
- ring-v-disk1a1.wm2d
- (28.53 KiB) Downloaded 52 times
Last edited by Fletcher on Sun Nov 24, 2024 9:11 pm, edited 1 time in total.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
daxwc wrote:I not so sure that is on solid assumption ground:Fletcher: "In every known example, his runners were firmly secured from floor to ceiling with sturdy support posts and bolts, presumably to limit 'sway.' What is inexplicable to me is that the runners were not placed in moveable crate/cradle supports resting on the floor, or put into a cart and via a belt drive making a self-moving cart, or a self-moving lake punt, for example, when turning a screw or paddle arrangement."
1)As additionally, he had a submarine prototype and a ship, which further complicates the understanding of his design choices. The complications of sway, waves, and water movement could significantly impact the stability and functionality of his designs, making it challenging to achieve the envisioned outcomes.
Hi dax .. when I said "known" it was in the context of published engravings or drawings in which we could see the external detail - these engravings etc were for the earlier wheels, not long on from the first runner prototypes ..
2)Also a boat would mean direction/orientation it sits seems to not be a total problem.
Who knows what improvements or changes he could make by then, perhaps mitigating early constraints to some degree - that's why I called it anecdotal evidence - I can only go on the published drawings and witnesses like Borlach ..
3) There was a table top version of a 3ft wheel. Why bother to say it sat on the table if it was secured at the roof.
Yes, the landlord's wheel many years later - IIRC it was called a "model" - if so, then it is conceivable that the small model did sit in a cradle ( perhaps of a size to sit on a table-top ) and could be broken down and stored for transport and put together again at a later time - and from that model, and following instructions, it was possible to make a larger more powerful wheel - and that wheel may or may not have been in a cradle support .. with no engraving or picture it's support method is only speculation ..
** I also found it beyond curious in the Merseburg translocation tests that it was not in a cradle support that could be slid or rolled on wheels along the floor to a new position, and back again etc - or, taken from one cradle and put into another one immediately beside it, and then the new cradle with wheel in-situ also slid or rolled to another position or compass heading - all we have in independent picture form is Borlachs engraving which shows supports bolted floor to ceiling ..
Also, our German friend here once mentioned he had read a publication that said a 10 inch final model was made - but he never gave any evidence of the publication - if is was true then clearly that was without a doubt a true table-top model - whether it was a working runner, in a cradle is not known, or a interesting facsimile that could be upscaled - whether just scaling it as it was would make it a more powerful runner, or some other variations were required can't be known without more reliable information ..
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
I don't see any impacts at the flag. I am talking impact energies at the flag differentials.
Last edited by daxwc on Mon Nov 25, 2024 12:10 am, edited 1 time in total.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Query: Objects with different "Moment of Inertia" rolling down a ramp will indeed accelerate to a maximum speed, but this maximum speed is not the same for all objects. The maximum speed depends on the object's moment of inertia, which is determined by the distribution of its mass; therefore all impact at the flags will have different impact energies due to v^2 acceleration component.
Copilot: Exactly! The different moments of inertia mean that each object will have a different maximum speed when it reaches the bottom of the ramp. Since kinetic energy is given by the equation KE=1/2mv^2, even small differences in velocity (𝑣) can lead to significant differences in kinetic energy due to the 𝑣^2 term.
Therefore, when these objects impact the flags, the energy involved in the impact will vary. Objects with higher velocities will have more kinetic energy, resulting in greater impact energy. This difference in impact energy is a direct consequence of the varying moments of inertia and the resulting differences in acceleration and velocity.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Flags on frictionless slides so they maintain their initial velocities received - both 'flags' weigh 10 kg each - both maintain separation and same velocity after contact indicating the same WD on both and have the same Translation KE .. imo ..daxwc wrote: I don't see any impacts at the flag. I am talking impact energies at the flag differentials.
................
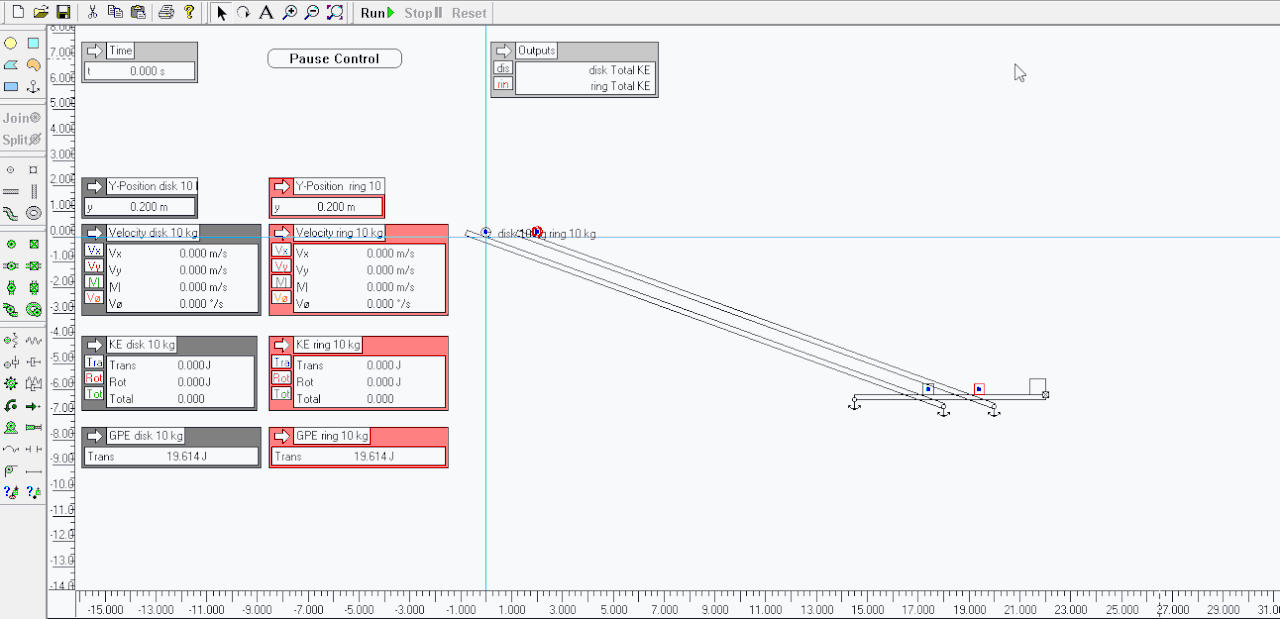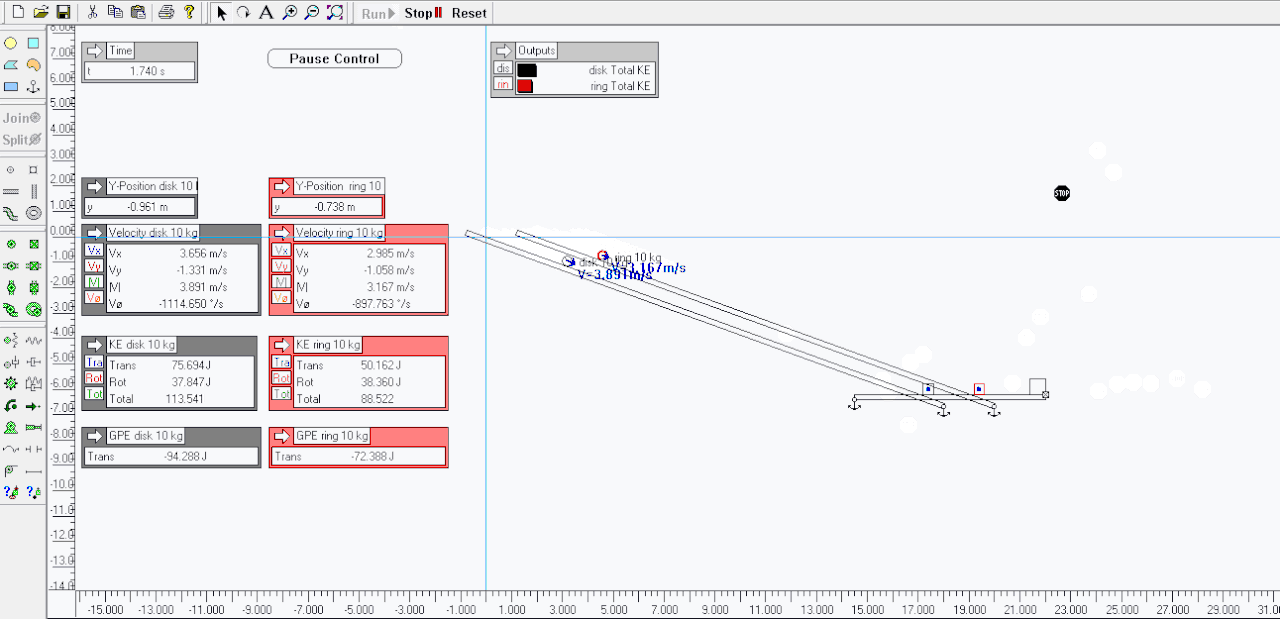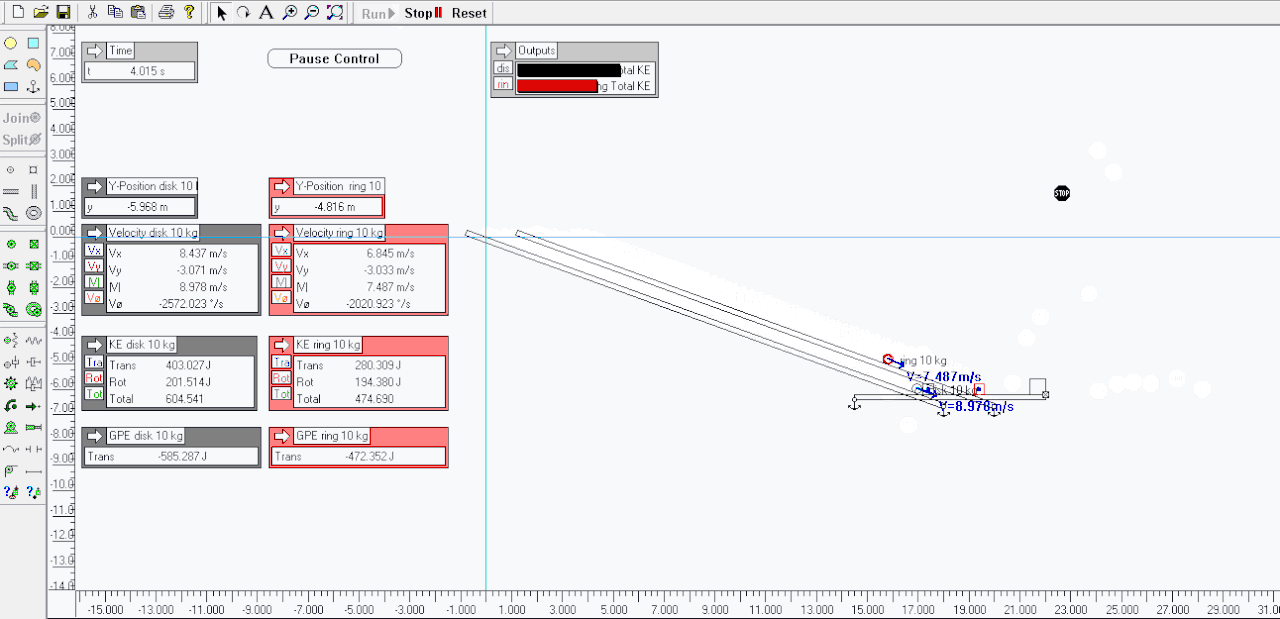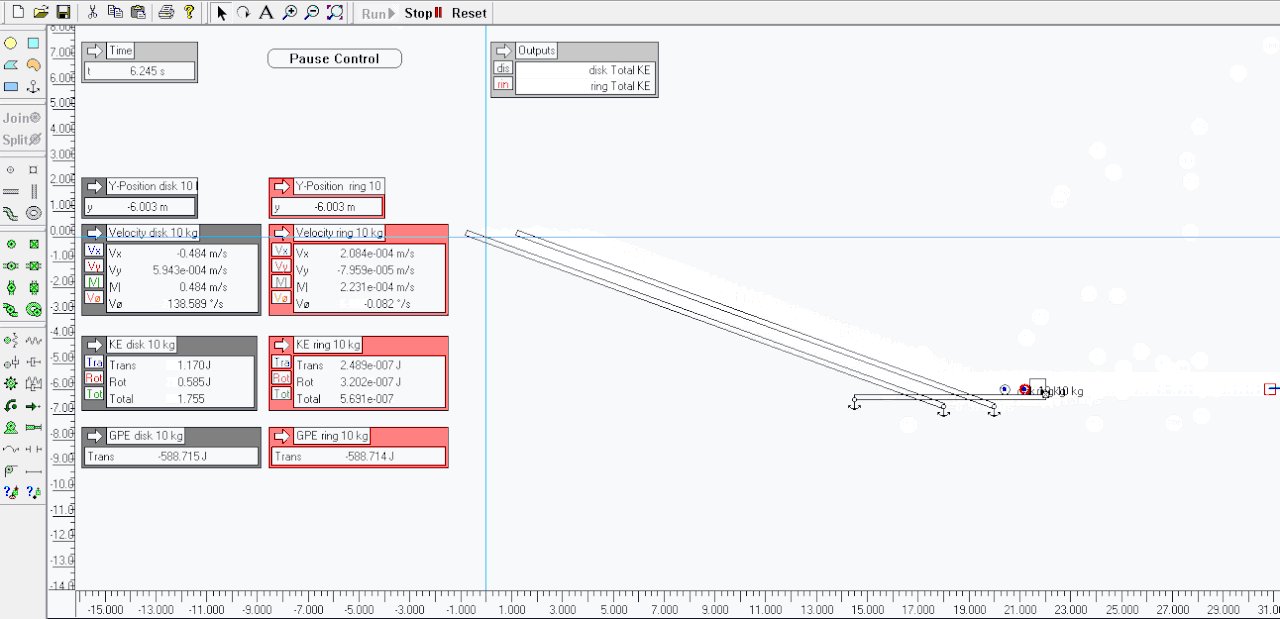
................
- Attachments
-
- ring-v-disk1a2.wm2d
- (30.93 KiB) Downloaded 52 times
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
The hoop is still rolling on its rim or shell. ROLLING! Not spinning rolling. Therefore it does the same distance down the ramp. So they are going the same distance but one has a formula in point mass that has a more negative energy (backwards distance per average point of mass component) slowing acceleration. So if the ramp gets very long and the rim on the hoop can not spin the more energy discrepancy due to different maximum speeds.
The way I see it anyway. I don't know how to explain it clearer.
Here is Copilots answer it seems to agree:
The way I see it anyway. I don't know how to explain it clearer.
Here is Copilots answer it seems to agree:
True. The hoop, while rolling on its rim or shell, covers the same distance down the ramp as any other object. The key difference lies in how the energy is distributed between translational and rotational forms due to its moment of inertia.
When the hoop rolls down the ramp, its kinetic energy is divided into translational kinetic energy (movement along the ramp) and rotational kinetic energy (spinning around its axis). The distribution of mass in the hoop, being further from the axis, means it has a higher moment of inertia. This results in a slower translational speed compared to objects with lower moments of inertia, like a solid ball.
At the bottom of the ramp, the hoop's total kinetic energy is a combination of its translational and rotational energies. When it impacts the flag, the energy involved in the impact will be different from other objects due to the
𝑣
2
term in the kinetic energy equation (
𝐾
𝐸
=
1
2
𝑚
𝑣
2
). This means that even though the hoop covers the same distance, its impact energy will be different because of its unique energy distribution.
In summary, while the hoop rolls the same distance down the ramp, its impact energy at the flag will differ due to the way its kinetic energy is divided between translational and rotational forms. This difference is a direct consequence of its moment of inertia and the resulting velocity at the bottom of the ramp.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Thanks Fletcher for all the effort.
I don’t know; doesn’t make sense to me.
Either the SIM is letting it spin up (not rolling on its rim)
Or
The max speed are exactly the same.
Both cant be true and have the same impact energy the longer the ramp gets.
I don’t know; doesn’t make sense to me.
Either the SIM is letting it spin up (not rolling on its rim)
Or
The max speed are exactly the same.
Both cant be true and have the same impact energy the longer the ramp gets.
Last edited by daxwc on Mon Nov 25, 2024 1:17 am, edited 2 times in total.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Coipilot: Simulation: If Fletcher's simulation shows the same impact energy for both the disk and the ring, it might be due to the specific parameters and assumptions used in the simulation. In reality, the impact energy should differ due to the varying velocities resulting from different moments of inertia.
In summary, if the hoop is rolling on its rim and not spinning in place, it will cover the same distance down the ramp as other objects. However, due to its higher moment of inertia, it will have a lower maximum speed and, consequently, a different impact energy compared to objects with lower moments of inertia. The simulation might not fully capture these nuances, leading to the observed discrepancies.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Just leave it. Your jerk example is way more easier to understand. Continue on. 8)
Last edited by daxwc on Mon Nov 25, 2024 1:47 am, edited 1 time in total.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
Well also odd if I remember right the table top model could not be brought out of the house or moved. Almost sounds like fraud.Fletcher: ** I also found it beyond curious in the Merseburg translocation tests that it was not in a cradle support that could be slid or rolled on wheels along the floor to a new position, and back again etc - or, taken from one cradle and put into another one immediately beside it, and then the new cradle with wheel in-situ also slid or rolled to another position or compass heading - all we have in independent picture form is Borlachs engraving which shows supports bolted floor to ceiling ..
I thought at one point he showed his wheel outside in Green Court due to the word shown at the "Fair". But as Bill pointed out it was at the fair but in his house.
Last edited by daxwc on Mon Nov 25, 2024 2:05 am, edited 1 time in total.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
I see it as this dax, fwiw .. the ring and disk have different inertia's - we all agree that the ring has more inertia than the disk because of its mass concentrated at the extremities, so it has greater resistance to being sped up or slowed down than the disk - if there is a set number of atoms for both ( have same mass ) each atom is rotated ( sine wave pattern ) around the objects traveling Center of Rotation ( as it rolls down the slope ) - iow's, the atoms of the ring on average have to move a greater circular distance as the ring rolls than those of the disk - to move a distance takes time, and each atom has the same individual inertia quotient in any direction .. thus altho both ring and disk rotate the same number of times ( ideal situation ) because the ring has the greater moment of inertia it can not have the same straight line velocity as the disk on arrival at its final height - meaning that both the ring and disk have the same Total KE - this is made up of Translational ( straight line ) KE and Rotational ( circular ) KE, but in different proportions because of their different moment of inertia from mass distribution ..
Therefore, when the ring and disk collide with the 'flag' both a portion of momentum and Total KE is transferred to the 'flag' n.b. momentum transfer is not often a complete transfer, especially when masses in collision are different, as can be seen by the original ring and disk continuing forward slowly after collision ..
An impact is a quick/sharp contact i.e. force applied for a short period of time ..
An impulse is a force applied usually over a longer time period compared to an impact ..
The relevance is that the disk gives most of its momentum and energy to the flag in what is more an impact scenario - most of that momentum and energy comes from the translational inertia of the disk as it has a higher velocity and a lesser angular inertia - a small portion comes from the rotating disk "followthru" i.e. it wants to continue rotating forward from ramp frictions and moment of inertia resistance to change in motion .. the ring collides with its flag with more of a prolonged impulse - it transfers its lesser translational KE and a higher proportion of "followthru" angular momentum and RKE to its flag - iow's it's moment of inertia and its friction contact with the ramp give it a greater followthru and time in contact - the sum of both straight line and followthru collision being more like an impulse than a traditional impact as physics describe them .. imo ..
Therefore, when the ring and disk collide with the 'flag' both a portion of momentum and Total KE is transferred to the 'flag' n.b. momentum transfer is not often a complete transfer, especially when masses in collision are different, as can be seen by the original ring and disk continuing forward slowly after collision ..
An impact is a quick/sharp contact i.e. force applied for a short period of time ..
An impulse is a force applied usually over a longer time period compared to an impact ..
The relevance is that the disk gives most of its momentum and energy to the flag in what is more an impact scenario - most of that momentum and energy comes from the translational inertia of the disk as it has a higher velocity and a lesser angular inertia - a small portion comes from the rotating disk "followthru" i.e. it wants to continue rotating forward from ramp frictions and moment of inertia resistance to change in motion .. the ring collides with its flag with more of a prolonged impulse - it transfers its lesser translational KE and a higher proportion of "followthru" angular momentum and RKE to its flag - iow's it's moment of inertia and its friction contact with the ramp give it a greater followthru and time in contact - the sum of both straight line and followthru collision being more like an impulse than a traditional impact as physics describe them .. imo ..
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
I suggesting that the simulation calculated the gravitational potential energy (GPE) for both the ring and the disk and ensured that their total kinetic energy (KE) just before impact was equal. This would mean that the simulation accounted for the conversion of GPE to KE as the objects rolled down the ramp.
What goes around, comes around.
Re: Hypothesis .. Raising GPE without using Law of Levers ? ..
I get it. You are talking about a total complete transfer of energy. Rather than an impact collision with a smaller mass; it is an impact impulse with a larger one.
Ok, Ok but that only works up to max speed. If you were at max speed at first 1/4 of the ramp where is this energy being stored for the last 3/4 ? After max speed the hoop has no where to store the energy and the faster velocity in the sphere begins to tally up.
Ok, Ok but that only works up to max speed. If you were at max speed at first 1/4 of the ramp where is this energy being stored for the last 3/4 ? After max speed the hoop has no where to store the energy and the faster velocity in the sphere begins to tally up.
What goes around, comes around.
