jim_mich wrote:The Eötvös effect was first brought to our attention by Debbie, one of the few gals to visit the forum.
Roland Eötvös (1848-1919) noticed that gravity readings were lower when on a boat sailing eastwards and higher when sailing westward.
From Wikpedia: "any object co-rotating with the Earth at the Equator has its weight reduced by 0.34 percent, thanks to the Earth's rotation."
The Eötvös effect is simply the change of gravity between an object moving East and one moving West. The Earth is moving at about 1040 MPH at the equator. Thus to take full advantage a weight must move backward (westward) at the same speed as the Earth thus eliminating all centrifugal force. Then the weight must do a U-turn and move forward (eastward) at the same speed on the Earth thus moving forward twice as fast as the Earth.
A 100 lb weight at the equator moving west at 1040 MPH will actually be moving at zero MPH and will weigh 0.34 lbs more than its 100 lbs. Conversely a 100 lb weight at the equator moving east at 1040 MPH will actually be moving at 2080 MPH. Because of the squaring effect of CF the weight will weigh 1.36 lbs less than 100 lbs. A 100 lb weight making a round trip down a ramp in a east direction and then back up a similar ramp in a west direction will have a 1.70 lb weight difference. The weight need not be solid. It can be water flowing in a pipe. The problem as I see it is that the 1.70% weight difference (at the equator) is not enough to overcome friction caused by movement at 1040 MPH.
Such a mechanism at a more northern location such as Michigan or New York City (about 45º north) will have about 70% as much power.
If friction could be kept to a bare minimum it might be possible to build a machine that gains enough energy from the Eötvös effect to run perpetually. It could be as simple as a tilted wheel spinning at a very high speed on magnetic bearings in a vacuum.
Or one might attempt to pump water back and forth along long sloped pipes, though again, I feel that friction would kill any potential gains.
Thanks for that contribution, Jim. It was so informative I have quoted it in full. It's invaluable having an addict with as good a memory as yours so that stuff can be dug up from the archives.
That bit about measurement on ships was certainly new to me - I don't think I was that clear of the difference between the Eötvös effect and the Coriolis effect before I had read the relevant link. It is fascinating to think that the difference can be measured at the relatively low speed of ships sailing east and west.
As far as "gravity assist" the original subject of this thread is concerned, I can now see that it was more of a lesson in how interactions between independent fields are discovered than a template for a gravity engine.
After all, even if the earth were not rotating or orbiting at all relative to the fixed stars it would still act as a sink for angular velocity wouldn't it.
Just as that sink extends indefinitely outwards beyond the earth (as was recognised by alexjrgreen earlier in this thread) so it must also extend indefinitely inwards to gravity itself. I am saying that the source of all angular velocity must ultimately reside in the gravitational field. That was the import of my little piece of doggerel which I repeat below.
Big whorls have little whorls,
Which feed on their velocity,
And little whorls have lesser whorls,
And so on down to gravity.
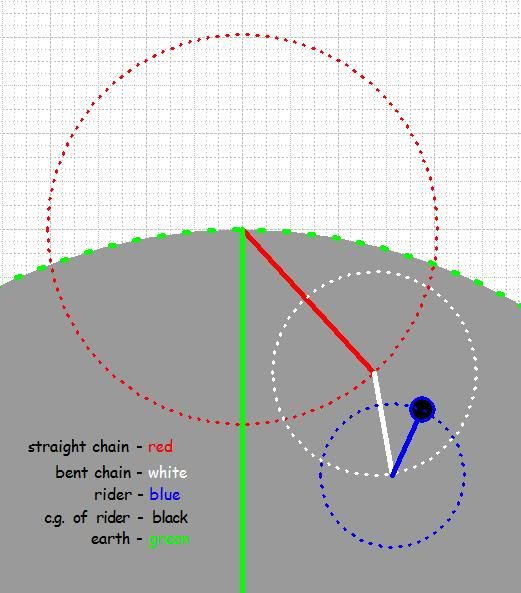
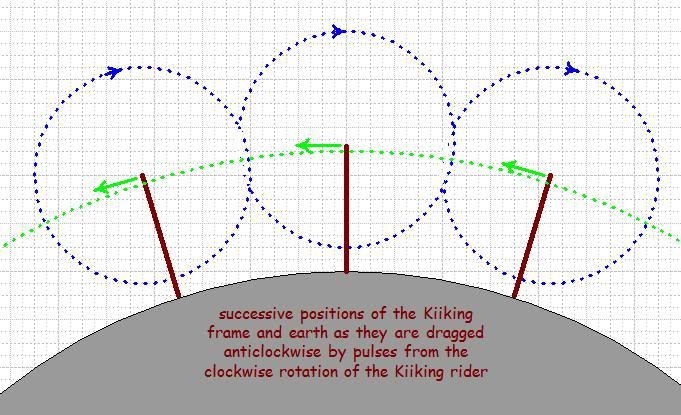
The human rider is just one possible location on the multiple paths of ascent between the angular rotations of the gravitational field and the angular rotation of the earth and beyond. The Bessler Wheel is another.
Needless to say I don't expect anyone to take this idea seriously until a gravity engine is built and Bessler is vindicated.
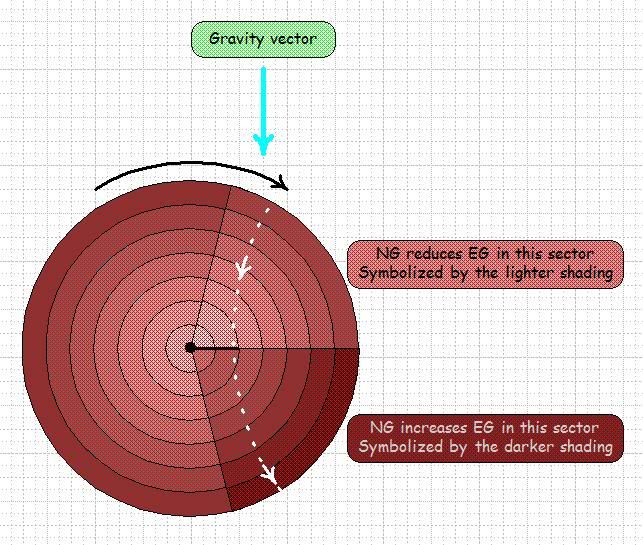
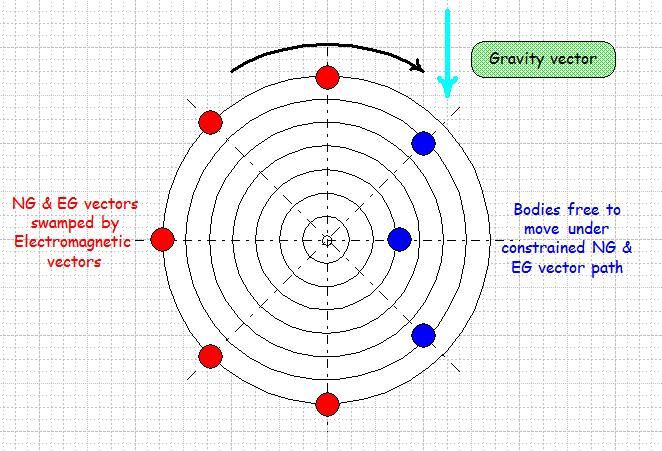
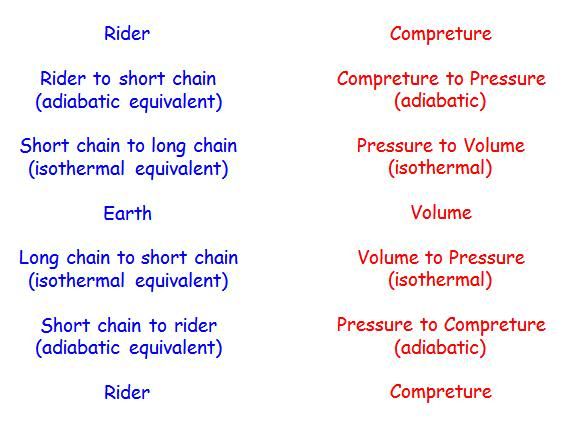
One can see the importance of Ersatz Gravity (CF/CP) in all this. It defines a universal wide spatial frame of reference. One might say that NG and EG are the alpha and omega of motion.