Buoyancy Wheel Design

[Bill_Mothershead]

In YOUR drawing...you are right!

But that is NOT the way I was thinking about it.

The beads I was thinking about were very small AND
not connected to each other. They are not a strand of pearls.

I see NO funnels in you drawing. This is to direct the unconnected
beads such that the all the floating or sinking power of the
other beads is concentrated onto just one bead.

Thought experiment: put a styrofoam brick in H20. Some of it
will be ABOVE the H2O waterline (=surface);

Next change the brick into tiny little beads. Put the beads in a
funnel pointed UP....then submerge the funnel till just the tip
is above the surface. The beads at the bottom will push up
on the beads at the top. SOME of the beads at the top
MAY come out...even if the tip of the funnel is somewhat
ABOVE the surface.

[Silvertiger]

This may be the last time I attempt to help anyone. I never know whether to give complex examples that go over peoples mental capacity, or to give very simple examples that leave out all the irrelevant bothersome details. I can do either. But I find it insulting when people tell me I'm wrong. True, I'm only human and do make mistakes, but to lecture me on basic concepts like mass density and such is just plain insulting.

I beg your pardon sir, but weren't you the one who asked if you had any errors? I apologize if I misread the message "Hopefully I've not made any typing or math errors ", but I pointed one out, which, to me, still remains erroneous. If you don't want anyone to politely disagree, then say nothing. To act that way is just an attempt to create drama, something I really don't like. Just play nice. It really doesn't matter to me how old you are as long as you act your age, because, simply put...I don't know you. If I did, I would know how to insult you. As far as "lecturing" goes, isn't that, hypocritically speaking, exactly what you did it to me with your "Let's do the math!" post? You assumed I was an idiot; so you posted something idiotic (no offense here, just perspective). But do you see me throwing a tantrum or sticking my nose in the air? If you cannot have a civilized conversation - and God forbid a disagreement - without getting angry or insulted, then simply post nothing. Problems are meant to be solved; not to be used as an excuse to act offended. That's not how I roll, and neither should you sir. So, you can either contribute, or you can go away. That's all. :)

Now, all that aside, I am a visual learner. If you truly do want to help, would you mind posting a free body diagram along with your resultant calculations so I can "see" what you are talking about?

[eccentrically1]

I agree with Jim; buoyancy is a balancing act.

[jim_mich]

Errors:
1. Density is not weight; its is the mass per unit volume of a substance. You multiplied a height of 5 by a density of 0.8g/cm^3,
but let's call it 800 since I prefer to work with kg/m^3.
Was the height you used 5cm, 5m, 5in...? Nevermind...let's call it 5m to keep units the same.

(5m)(800kg/m^3)=4000kg/m^2

What you have effectively done here is defined BMI for some reason, a calculation primarily used in sports and medicine.
Whomever this represents is clearly the most obese creature in the world.

Until this is addressed, I won't even attempt a guess at anything else. :)

My use of specific gravity ratio is not a "typing or math" error. It is done on purpose. Initially you did not give any volume data. Volume data is not needed to analyze this situation. All that is needed is the specific gravity ratio. Also specific height data is not needed to analyze this situation. All that is needed is the ratios between the two liquid columns.

The whole situation can me reduces to one single buoy as it makes one full circuit.

Choose any volume desired for the buoy. Let's say the buoy is the size of a tennis ball, 2.625 inches or 66.675 mm. This volume pi÷6×2.625^3 = 9.45 cubic inches
Water has a density of 62.3 lbs per cubic foot at 71ºF (room temperature), or about 0.576852 oz per cubic inch. So the weight of water the size of a tennis ball will weigh 5.46325 ounces. Now assume we fill the tennis ball with some substance which causes the tennis ball to weight 90% of water, the filled tennis ball will weigh 4.9167 ounces. It will displace 5.4632 ounces of water and will displace 4.3706 ounces of oil.

When in oil, the filled tennis ball will exert 0.5463 ounces of downward force and when in water it will exert the same 0.5463 ounces of upward force.

Now we need to determine the desired column height. You suggested 5 meters (16.4 ft). I think this height is ridiculous for testing purposes, so let's use something more realistic, say 50 inches (1.27 m) of oil, which will support 40 inches (1.016 m) of water.

The buoy will sink 50 inches at a downward force of 0.5463 ounces, thus it produces 50 × 0.5463 = 27.3162 inch/ounces of work while sinking.

The turn around at the bottom is equally down as up, so we can ignore it, since the turn around forces balance.

The buoy will rise 40 inches at an upward force of 0.5463 ounces, thus it produces 40 × 0.5463 = 21.8530 inch/ounces of work.

The two forces combined produce 27.3162 + 21.8530 = 49.1692 inch/ounces of work

The buoy needs to rise another 10 inches from the top of the water to the top of the oil. The buoy weighs 4.9169 ounces. So this movement will require 10 × 4.9169 = 49.1692 inch/ounces of work.

The final turn around at the top is equally up as down, so we can ignore it, since the turn around forces balance.

The work force needed to raise the buoy from the top of the water to the top of the oil (49.1692 inch/ounces) exactly balances the work force (49.1692 inch/ounces) produced by the sinking and rising of the buoy.

So, I've used real numbers and real values in a realistic scenario, proving that the system balances. You can change any of the dimensions and sizes to suit your particular situation, but it will still always balance.


As far as I'm concerned, using actual number values makes it much harder to follow the logic of what is happening. It is simpler to envision a weight of 0.9 specific gravity (+0.1 relative specific gravity to oil and -0.1 relative specific gravity to water) sinking in oil a relative distance of 5 while rising a relative distance of 4 thus producing a relative work force of 0.9 which balances against the relative work force needed to lift the 0.9 specific gravity weight back up the 1.0 height from the top of the water to the top of the oil.

[Tarsier79]

Jim, I can say your calculations are appreciated by at least some.

Silvertiger, first read http://en.wikipedia.org/wiki/Buoyancy

"Buoyancy = weight of displaced fluid." And as you read further, you will notice density as part of the equation.

The weight of the displaced fluid is due to gravity, therefore buoyancy is merely an equal and opposite reaction to gravity. As your buoy moves up, fluid weight moves downwards in the system, and the fluid surrounding your device is included in that system. An equilibrium of forces through a full rotation is not enough to create a sustained motion.

Thanks for posting your experiment.

[Silvertiger]

Silvertiger, first read http://en.wikipedia.org/wiki/Buoyancy

"Buoyancy = weight of displaced fluid." And as you read further, you will notice density as part of the equation.

I took basic physics quite some time ago. I'm pretty sure that Archimedes' discovery hasn't changed in that time lol. :)




So...Jim. I'll start off with some knowns. :)

W = pVg; V = (4pir^3)/3; p[H2O] = 1000kg/m^3; Radius of Tennis Ball = 0.0335m


Calculations:

We fill the tennis ball to make it 10% less dense than water, so:
p[TB] = 900kg/m^3

V[TB] = (4pi0.0335^3)/3 = 1.5748E-4m^3

W[TB] = (900kg/m^3)(0.00015748m^3)(9.81m/s^2) = 1.3904N

W[H2O] = (1000kg/m^3)(0.00015748m^3)(9.81m/s^2) = 1.5449N

W[Oil] = (800kg/m^3)(0.00015748m^3)(9.81m/s^2) = 1.2359N

Buoyant force acting on TB in H2O:
F[B[TB[H2O]]] = W[H2O]-W[TB] = 1.5449N-1.3904 = 0.1545N

And in oil:
F[B[TB[H2O]]] = W[Oil]-W[TB] = 1.2359N-1.3904N = -0.1545N



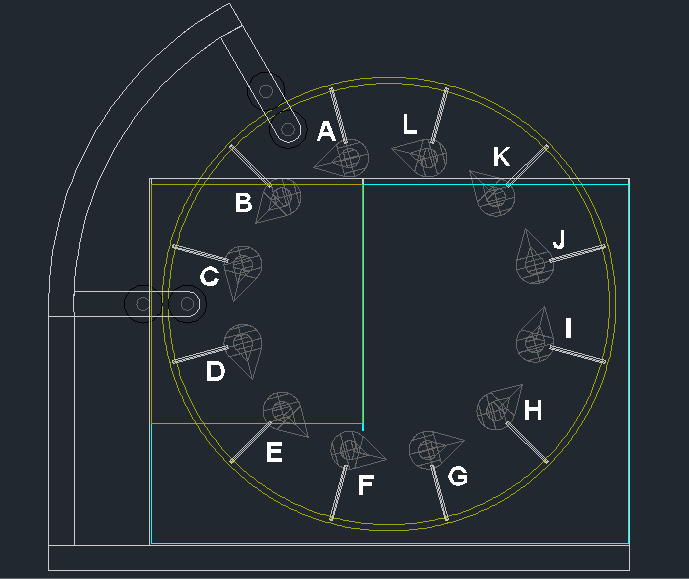
In this drawing, assuming that what we're seeing are the filled tennis balls, weight F will balance with G, and A will balance with L, but AL will also have a top-heavy advantage over FG as well. Aside from these balances, however, there will be an imbalance of forces between BCDE and HIJK. BCDE will have a net downward force, or weight, of -0.618N, and HIJK will have a net upward force of 0.618N. The net linear force imbalance (aka potential difference) between them would then be 1.236N, resulting in a rotation powered by over 1N of tangential force. Tell me if I missed anything.

[Tarsier79]

What about the difference in fluid height between the two liquids? If you look back to your experiment, the lighter fluid is lifted to a higher level at the top. This is the section that will balance your figures. B and K will be your problem.

[Silvertiger]

That's why weights F and G balance - because they are both submerged in water and the buoyant force acting upon them is identical in both magnitude and direction, assuming that the wheel is just sitting in this position and is not rotating. The difference in height between the fluids across B and K will be negligible - a minor hair's breath in loss of force at best. Instead of the potential difference being 1.2N, it might be, say, 1.09 or something like that. But it would never negate the potential difference that exists. And as long as there is an imbalance in the apparent weight, rotation can and must occur. The height in the oil won't matter too much because the weights will never rise in it. To be honest, the biggest problem I can foresee is the possibility that a significant amount of oil would cling to the weights and over time get equally distributed on both sides and even out the fluids. That would be bad.

[Tarsier79]

If you have a balanced see saw made of lead, and submerge half in water, what will happen? It doesn't matter that the lead is heavier than water.

[Silvertiger]

Give me a minute on this one.

[Tarsier79]

Also, try calculating the height difference in the liquid in your proposed disign, then multiply that by the buoyancy force. Hopefully you will have some interesting results:)

[Silvertiger]

I'll have to generate some givens:

All downward forces, such as gravity, will be assigned negative values; all upward forces positive values.

Seesaw board dimensions:
H: 0.05m
W: 0.5m
L: 5m

Density of Lead: p[PB] = 11,360kg/m^3

Volume of lead board: V[PB] = (0.05m)(0.5m)(5m) = 0.125m^3

Let's assume that exactly half of one side is submerged.
V[PB[submerged]] = (0.125m^3)/4 = 0.03125m^3

Weight: W = pVg

W[PB] = (11,360kg/m^3)(0.125m^3)(-9.81m/s^2) = -13,930.2N

W[1/4PB] = (13,930.2N)/4 = 3,482.55N

W[H2O] = (1000kg/m^3)(0.03125m^3)(-9.81m/s^2) = -306.5625N

Buoyant Force: (aka apparent weight)
F[B[PB]] = W[PB] - W[H2O] = -13,930.2N - (-306.5625N) = -13,623.6375N

It's apparent weight will become -13,623.6N in water.

Of course, it could only submerge if one side was heavier. But the end result is that the submerged side will decrease the overall apparent weight of the board and thus change the angle that it sits at.

[Tarsier79]

Sorry, I meant submerge just one side in water. (oops should have read your post properly)

The side submerged in water will float.

The height in the oil won't matter too much because the weights will never rise in it.

It will rise in it, due to the opposite weight being in air. The oil provides a buoyancy force even though it floats on water.

[Silvertiger]

Also, try calculating the height difference in the liquid in your proposed disign, then multiply that by the buoyancy force. Hopefully you will have some interesting results:)

I don't really have to calculate it. Let's say that the difference in height was enough to completely submerge weight A in oil. You would still have the combined downward force of of BCDE and the buoyancy of HIJK to act against it, as well as against L. There would still be a potential difference against those weights.

[jim_mich]

Tell me if I missed anything.

What about the difference in fluid height between the two liquids?

The difference in height between the fluids across B and K will be negligible - a minor hair's breath in loss of force at best.

Also, try calculating the height difference in the liquid in your proposed disign

I don't really have to calculate it. Let's say that the difference in height was enough to completely submerge weight A in oil. You would still have the combined downward force of of BCDE and the buoyancy of HIJK to act against it, as well as against L. There would still be a potential difference against those weights.

The difference in height between the top of water and top of oil is calculable. It is relative to the density difference between the water and oil. If the specific gravity of the oil is 0.8 then the top of the water will be 0.8 times the oil height.

You cannot ignore the height difference between the top of the water and the top of the oil. Raising the buoys from the top of the water up to the top of the oil WILL NEGATE all the force gained by the buoys as they travels through the oil and water. This is the bottom line. This is what I've been saying in each of my postings in this thread.

The sinking force is only 1/10 of the weight of water. And the rising force is also only 1/10 of the weight of water. But when you raise the weight upward from the top of the water to the top of the oil, you must raise the full weight of the buoy, which is 9/10 of the weight of water. And this height from the top of the water to the top of the oil is 0.2 times the full height of the oil. When all is calculated then everything balances.