In order to crack Bessler's code I had to think like him and solve problems the way he would solve them. In many ways I had to become Bessler. In this thread I will attempt to resurrect Bessler's mind and his wheel.
The first and greatest clue to cracking Bessler's code is to look at his background. Bessler was a clockmaker by trade so naturally he saw his wheel as a clock. His wheel was built like a clock.
The directions in the boards in his drawing almost hint at a clocklike mechanism. The Bessler Wheel is actually two clocks: the larger wheel itself and a series of clocks acting as hands on the main clock. The clocks are sycronized to three O'clock and move in such a way as shown in the diagrams below.
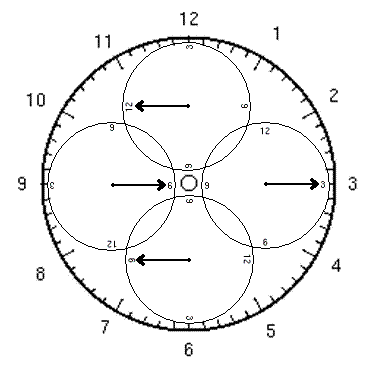
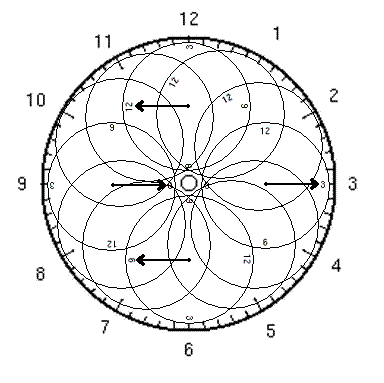
The movement of these clocks creates what is known as a sacred geometry. Sacred geometry is an ancient knowledge with differnt rulesets than Euclidian geometry. Sacred Geometry allows for over unity leavers and various squared circles. Sacred Geometry has had a role in everything from alchemy to building the pyrmids and can even be found in the structures of living organisms and the perpetual rotation of the planets. It is unknown if Bessler independently discovered sacred geometry or read about it in surviving alchemical texts of the period.
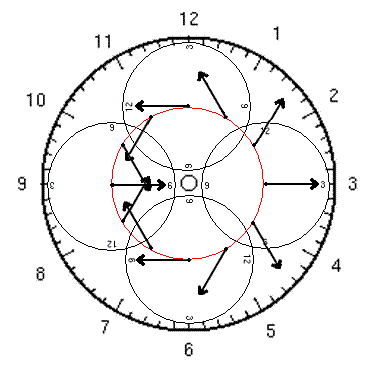
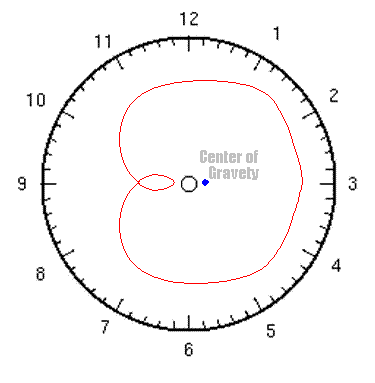
On the tip of each arrow would be a heavy weight. Using this geometry the weights are moved up the center of the wheel where they have little mechanical advantage. While going down the weights would travel down the wheel's edge where they have exponentually greater mechanical advantage. This wheel if built this way would have a perpetual off center gravity which would cause it to rotate and rotate indefinately. The continual movement of the machinery keeps the wheel pretetually off balance as the wheel turns. The power of this wheel is exponentialy proportional to its diameter. Longer diameters bring the weights closer to the center axis while rising and at the same time farther from the center while falling.
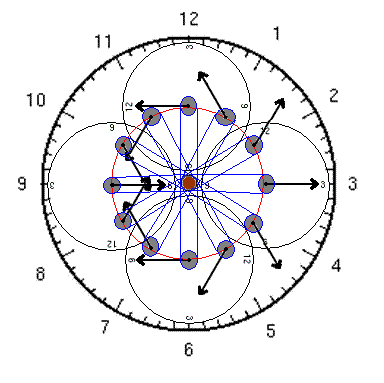
Implementation of this complex geometry is remarkably simple. A single stationary gear in the center powers all the other weights. As the wheel turns a chain indepentently turns each arrow. Each complete rotation around the center axis will turn the arrows exactly once. These drawings account for 12 weights but Bessler may have used 16 or even 32 which create greater over unity sacred geometries.
So now that I have shown you how Bessler built his wheel the race is on to see who can build this rather simple clockwork first.





 <-- I'm with stupid
<-- I'm with stupid