
Posted by Scott Ellis (216.87.95.64) on March 14, 2002 at 00:50:32:
There are many dynamic physical principles that can cause unusual effects in mechanical systems. If Bessler was genuine, he probably exploited one of these principles. One obvious example is the gyroscopic effect, where the apparent weight of a mass is offset from it's gravitational center if the mass is allowed to precess as it spins.
I would like to describe 2 other cases where I think an unusual but natural, dynamic principle will cause actual results to differ from the intuitive expectation.
Regardless of their merit, I hope you will keep these ideas in confidence, and I hope you will provide me with feedback about them.
The two ideas are:
1. The net vertical distance gained in the resonant case of the oscillating spring pendulum
2. The impact vs. impulse nature of collisions of solid bodies
I will describe each idea in detail:
1. Parametric Excitation of Oscillation in the Spring Pendulum
We all know the rule: Rolling a ball in a bowl, swinging a pendulum, you can never get higher than you started. And due to friction, you can't even break even.
But in truth, we can raise a weight by a certain vertical distance X, release it, then catch it at a height of 3X or more!
A metal ball hangs on a spring, which is fixed in turn by one end to a board. If we pull the ball down and release it, the pendulum will oscillate in the vertical direction and then it gradually begins to swing from side to side similarly to a regular pendulum. After a while the horizontal oscillation will cease and vertical oscillation will arise again. The system will behave the same way if the ball is deviated aside and released. In the resonant case, the transfer of energy from the horizontal oscillations to the vertical oscillations is maximized.
The key to maximizing the resonance is this formula:
mg 1
-- = -
kl 4
http://www.maths.tcd.ie/~plynch/SwingingSpring/System.html
Now check out this link:
http://www.maths.tcd.ie/~plynch/SwingingSpring/swingingspring.html
The applet shows how a resonant swinging spring transfers energy from horizontal oscillations to vertical oscillations. What occurred to me is this: When analyzing the spring-weight system, every point on the path represents the same energy (spring energy + kinetic energy + gravitational potential = CONSTANT), that is true enough. But from a reference point totally outside the system, placing the weight on different points on the path represent very different energies. In particular, the path seems to transform horizontal distance (which is basically free with low friction), into upward vertical distance (anything but free). The pendulum goes higher than we started:

From a reference point outside the system, it looks like we can raise a weight by a small vertical distance (real work) and push it over a larger horizontal distance (basically free), and end up with the weight at a position that represents substantially more (potential) energy than we started with:
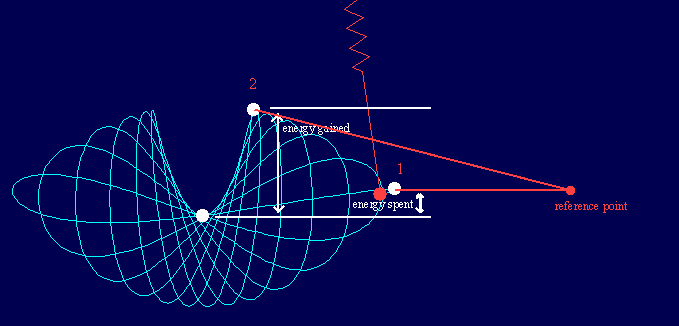
If the weight were actually attached to a lever arm pivoting at the reference point above, the two (supposedly equal) positions of the weight would represent substantially different energies. If the weighted lever arm marked 2 were released it could do MUCH more work (> 2x) than the arm marked 1. (The difference would be even greater if we caught the weight at the apex point on the left)
???
----------------------------------------------------------------------------------------------------------------
2. Impact vs. Impulse and Inertial Thrust
Imagine 2 weights on a wheel are always perfectly balanced. They must alwa the paper Davis puts forward, rather convincingly, some strikingly persuasive arguments for the possible existence of a force proportional to the third time derivative of displacement (or position), usually known in engineering circles as "surge", "jerk", or "rate-of-onset."
Below are some important extracts.
The class of anomalous behavior which we wish to study involves in every case the presence of surge as we have just defined it. Under conditions of constant or zero acceleration mechanical bodies or systems of bodies obey Newton's Laws reasonably well. It is under conditions of changing acceleration that difficulties arise. The key word in our analysis of dynamic systems will be "simultaneity". The Laws of Motion presuppose exact simultaneity of action and reaction......to satisfy Newton's image of the universe.The following year (January 1935), an article appeared in the magazine 'Popular Science', showing some drawings depicting the basic unit and a photo of the author flipping the switch on a pendulum mounted set-up of the unit. These are some extracts from the Popular Science article;Newton's Laws, strictly speaking, apply only to mathematically infinitesimal particles or perfectly rigid bodies, neither of which exist in the real world.
Consider, for example, a simple steel rod one meter long which I wish to move by applying a force to one end. The instant I start to apply the force a message leaves the end of the rod as a plastic or elastic compressive wave which travels at a speed of approximately, 5,000 meters/second. The compressive wave travels to the far end of the rod where it is reflected as a rarefaction and returns to the point of application of force at the same speed.
Until the wave returns, 4/10,000 of a second later the rod as a whole cannot move according to the Second Law! No matter how much force is applied, the center of gravity of the rod cannot obey F = ma in less than this time. It would be oversimplifying to say that the rod acts as though it had infinite mass during this time, since the center of gravity will be moved somewhat by the compression, but for all practical purposes, the rod acts as though it had a much larger mass than it actually has.
...The particular behavior of a given system will depend on: (a) how rapidly the force is applied and, (b) the built-in delay time, or "critical action time" of the system. More exactly, we have found that the behavior depends upon how rapidly one attempts to change the acceleration applied to the body. The ultimate acceleration of the body, the "a" of F = ma, is not what is critical; it is the rate of onset or "surge" of the acceleration which is vital.
...There is no conflict here with Newton, for Newton considered only systems where either velocity or acceleration were constant. Since his data inputs were from astronomy and since he had no instruments capable of investigating effects of changing acceleration - effects that may occupy milliseconds or less - this is not particularly surprising.
...Starting transients normally are considered only in connection with the beginning or the end of a motion and hence are accorded no particular attention. However, there are certain types of cyclic motion where the transient behavior is continuous, or repetitive and we will see later that even certain single transients may have critical importance in understanding natural events...[This] solution demonstrates the most significant characteristic of real bodies, to wit: not only is displacement somewhat less than Newton would predict for a given force, leading to an increased apparent mass, but reaction is no longer exactly opposite to the applied force: there is a phase angle which will be larger the longer the critical action time of the system. Action and reaction are not simultaneous.
We come to a consideration of what must be the Fourth Law of Motion. There will obviously be several alternative expressions. Mathematically, what seems to be critical in systems with intractance is the rate of change of energy, so that the Law is perhaps best expressed in these terms:
The energy of a given system can only be changed in some finite length of time depending on the system, and never in zero time.
... At this point, it is proper to ask th inch diameter steel rod. The chambers were arranged to explode in series, blowing the steel disks downward with great velocity. Knowing the weight of the disk and its velocity and also the weight of the complete rocket it is a simple matter to find the energy of the recoil. Although this type of rocket works, it leaves much to be desired in the line of safety.
A series of tests now followed which I believe are original in the field of reaction. They dealt with a form of impact-impulse reactions which are created mechanically. The apparatus if placed in a box in space would move without the use of a jet of any sort, it being propelled by a reciprocating motion of two weights. The truth of the theory may be easily proven by a very simple apparatus.
The theory is that a large weight with a low velocity ( if stopped by springs ) will yield more foot pounds of energy than a small weight with a high velocity being stopped by impact, even though both were given the same initial force. A simple illustration: Let us assume you are in the center of a room in space. One wall is elastic and the opposite one solid. In your hands are two balls one heavy and the other light and you throw them with the same force at the same time. The heavier one hits the elastic wall with an impulse and a large amount of energy is given to the room in that direction. The lighter one having the same energy hits the solid wall but its energy is dissipated in heat and distortion. If there could be found a method whereby the kinetic energy of the lighter ball could be effectively utilized the room would move in the other direction.
This elementary form of reaction motor operates on a principle that has long been neglected by engineers, but which Bull believes can be applied in aircraft and other vehicles. It depends upon the difference in effectiveness of two ways of transmitting energy, which can be termed impact and impulse. If a weight is thrown against a solid wall, it is stopped by impact, and much of its energy is wasted in distorting the weight and the wall and in producing heat. However, if the weight is thrown against a spring fastened to the wall, it is stopped by impulse, the spring conserving the energy of the moving weight and transmitting the resulting force, with little loss, to the wall. Tests have shown a weight will yield three times more force by impulse than by impact.This is perhaps the simplest proof of concept device imaginable. Indeed the device works as claimed. The only important question that remains is, if the cycle can be closed and made continuous (i.e. repeatable) without affecting its generality. As it stands the device acts as a 'one-shot'. That is, it requires resetting before each demonstration....Achieving a practical reaction motor, Bull points out, depends to a large extent, paradoxically, upon how inefficient it can be made. The more force that can be wasted in impact, the greater force will be left to push ahead, a new problem for engineers, who have spent years trying to conserve energy rather than dissipate it. Likewise, much experimentation remains to be done upon the impulse side of the apparatus, which is still far from efficient.
Here is the source page for all of the above information. If you have time, it is worth reading the whole thing: http://www.google.com/search?q=cache:7NhUqw17c50C:www.motordyne.com/evidence.htm+impulse+impact+inertia+thrust&hl=en
Here are some more links on inertial propulsion devices:
http://jnaudin.free.fr/html/impdexp.htm